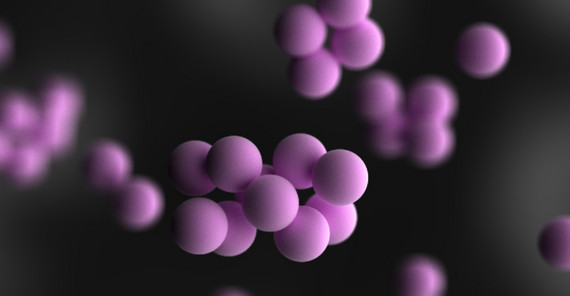
Self assembly is an important process in which simple building blocks aggregate into a larger more complex structure. A simple building block of continuing research interest are hard spheres, which are of interest to mathematicians and physicists alike, who use hard sphere models to model atoms, colloids and liquids. As early as 1611, Johannes Kepler suspected that the densest way to stack hard spheres was in a pyramid shape.
For a paper recently published in the ‘Proceedings of the National Academy of Sciences’ (PNAS) the mathematician Ivan Spirandelli and his team modeled a solution in which a hard sphere solute interacts with a hard sphere solvent. The model of solvation they utilize depends on the geometry of the arrangement of solute particles. “For many of the systems we see arrangements that have previously been observed. In other parts of the considered parameter space, however, we find exotic structures such as double helices, rhombohedra and barrel like structures surrounding a central spine” he describes. These intricate and exciting structures highlight the importance of geometric considerations in self assembly settings.
Ivan Spirandelli, who is a PhD student in Applied Geometry and Topology at the Institute of Mathematics, has further been successful in obtaining a prestigious Fulbright fellowship for PhD students. He will have a research stay at the Lawrence Berkeley National Lab in Berkeley, USA, where he will work together with Dr. Dmitriy Morozov on a joint project on persistent homology and topological optimization in morphometric simulation.
The Fulbright Program, founded in 1946, promotes international educational exchange to enhance mutual understanding between nations. Sponsored by the U.S. Department of State's Bureau of Educational and Cultural Affairs, it supports scholars, students, and professionals through grants for research, teaching, and cultural experiences abroad. By fostering academic collaboration and cross-cultural dialogue, Fulbright contributes to global cooperation and connectivity.
Link to paper: Ivan Spirandelli, Rhoslyn Coles, Gero Friesecke, and Myfanwy E. Evans, Exotic self-assembly of hard spheres in a morphometric solvent, PNAS 2024, https://doi.org/10.1073/pnas.2314959121
Contact: Ivan Spirandelli
Institute of Mathematics, Applied Geometry and Topology
E-Mail: spirandelliuuni-potsdampde